 SNOW HYDROLOGY (GEOG 4321): Last week notes
SNOW HYDROLOGY (GEOG 4321): Last week notes  SNOW HYDROLOGY (GEOG 4321): Last week notes
SNOW HYDROLOGY (GEOG 4321): Last week notes
powerpoint: Meltwater Flow Through Snow
Movement of liquid water through snowpacks is one of the least understood aspects of snow hydrology [Richter-Menge and Colbeck, 1991]. It has an important influence on the timing and magnitude of snowmelt hydrographs [Caine, 1992] and on biogeochemical and geomorphological processes [Williams and Melack, 1989; Caine, 1995]. Adapting more physically-based approaches to understand and model flow through a snowpack should permit wider applications of operational snowpack models to more sites, and allow for year-to-year variability within a site [Melloh, 1999]. Similarly, research on glacial hydrology has shown that the least-understood part of this system is the simplistic way that current models treat meltwater storage and routing through supraglacial snowpacks [Arnold et al., 1998].
A considerable amount of research has been undertaken to investigate the underlying physics controlling water movement through snowpacks [Colbeck, 1972, 1973; Ambach et al., 1981; Jordan, 1983; McGurk and Kattelmann, 1988]. Results have revealed a complex series of processes involving grain growth, snowpack thinning, water flow through variably saturated porous medium, and refreezing of meltwaters within the snowpack, all of which act to change the hydraulic and thermal properties of the snowpack [Kattelmann and Dozier, 1999]. These studies have also shown that snowpacks are highly heterogeneous due to microscale variations in density and ice grain structure, combined with macroscopic layering [Colbeck, 1991]. However, few experimental studies have been conducted to quantify these processes under natural field conditions since Colbeck [1975] remarked on the need for more such studies in the early 1970's.
Movement of liquid water through snowpacks is generally recognized to occur in distinct flow paths rather than as uniform flow through a homogeneous porous medium. Here we treat as synonyms the terms flowpaths, preferential flowpaths, flow channels, pipes, macropores, and flow fingers. Seligman [1936] found that snowpack permeability was enhanced when flow channels were present in the snowpack. Oda and Kudo [1941] described flow fingers and flow along layer interfaces. Dye was used to trace flow paths during the Cooperative Snow Investigations [Gerdel, 1948; 1954; US Army, 1956]. Ice columns, described previously by Ahlmann and Tveten [1923], Ahlmann [1935], Seligman [1936], and Gerdel [1948], were recognized to be the residual flow network in cold snow by Sharp [1951]. Grain growth in paths of preferential flow was related to higher permeability and more efficient flow [Wakahama, 1968a]. Saturated conditions and non-Darcy flow were also observed in these flow paths [Wakahama, 1968b]. Heat transfer and alteration of snowpack structure by freezing during infiltration have been investigated by Colbeck [1976], Illangasakare et al. [1990], and Pfeffer et al. [1990, 1991]. Zones of preferential flow and ice columns have been observed in many other studies [e.g. Wankiewicz, 1976; Jordan, 1978; Denoth et al., 1979; Higuchi and Tanaka, 1982; Marsh, 1982 and 1988; Kattelmann, 1985 and 1986]. However, attempts to characterize the spatial distribution of preferential flowpaths have had only limited success [Marsh and Woo, 1985; Kattelmann, 1989]. Attempts to understand two-dimensional meltwater flow through snow from first principles have also had only limited success [Colbeck, 1979; 1991]. Overall, our ability to understand the spatial distribution of preferential flowpaths in melting snowpacks has suffered from the ephemeral nature of the flowpaths and the problems caused by destructive sampling of the snowpack (e.g. Schneebli [1995]).
Our collective research endeavors have led us to believe
that a new approach must be developed in order to
achieve significant advances in our understanding of
meltwater flow through snow at the grain scale.
Modeling the movement of water through snow
has generally followed the approach of Colbeck [1972],
in which the effects of capillary pressure gradients are considered
negligible,
yielding the simplified form of Darcy's equation
[Albert and Krajeski, 1998].

but has resulted in
current models of meltwater flow through snow,
such as SNTHERM [Jordan, 1991] and
SNAP [Albert and Krajeski, 1998], that are limited
by inadequate characterization of parametric functions
for water retention in snow under unsaturated conditions.
Relative permeability has traditionally been written
as an empirical power function of effective saturation
[Corey, 1986]

Both epsilon and kappa sub rw depend upon physical properties
of the snowpack,
and may change considerably
during the melt season as snowpack metamorphism affects grain size,
density, and ice layer thickness
[Marsh, 1991].
Denoth et al. [1979] measured values of @epsilon@ that ranged
from approximately 2 for new snow to 5 for old snow;
whereas Colbeck and Anderson [1982] and Jordon [1983b]
suggest using an integer value of 3.
However, previous work has not been able to establish any
relationships between
epsilon
and kappa sub rw and snowpack properties or amounts of metamorphism
[Denoth et al., 1979].

Several methods are available for estimating parametric functions
(capillary pressure, saturation, hydraulic conductivity)
of water retention under
unsaturated conditions.
Basically, there are three formulations to represent
the capillary pressure-saturation function (or retention model):
(1) Brooks and Corey's model
[Brooks and Corey, 1964;
Corey, 1994];
(2) van Genuchten's model
[van Genuchten, 1980]; and
(3) the exponential model
[Gardner, 1958; Russo, 1988].
For snow,
observed data are generally not available and
semi-empirical equations are used to estimate
hydraulic conductivity
versus saturation or capillary pressure.
Essentially,
the van Genuchten approach is used to determine
kappa by substituting the capillary-pressure and effective
saturation of Brooks and Corey [1964]
into the statistical model of Mualem [1976].
Mualem's model [Mualem, 1976] uses a fitted
capillary pressure-saturation function to derive the
relative hydraulic conductivity (Kr)
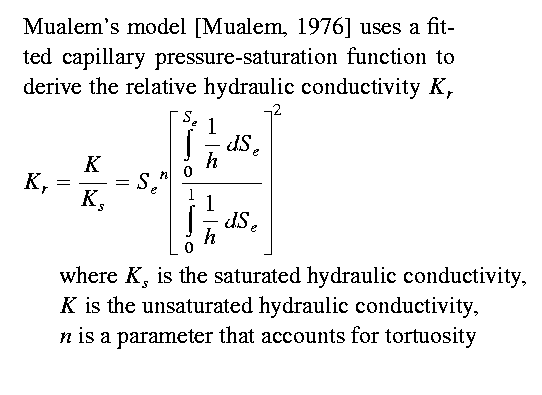
Mualem's model assumes that in different pore segments of an unsaturated porous medium, the flow occurs as pipe flow. Certain inter-connected pore spaces transmit water in the form of fully saturated pipes, while other pores remain empty. Based on data from field soils with a high clay content, @n@ was approximated to be 0.5 [Mualem, 1976]. While the Mualem model provides a good approximation of water flow through snow [Illangasekare et al., 1990; Tseng et al., 1994a], we do not believe that the Mualem model of fully saturated pipes accurately represents the physical processes in a snow pack and that a new paradigm for liquid water movement through snow must be developed.
The properties of snow cannot be directly measured over entire hydrologic basins because of cost, labor, access difficulties, and destuctive sampling techniques. Consequently, measurements obtained over small areas and at point locations must be extrapolated over large areas. Geostatistics provides a framework for performing this extrapolation, and for modeling the uncertainty that is inherent in the estimates produced by extrapolation. Geostatistics also provides a framework for combining data obtained at different scales and varying levels of accuracy, such as field measurements vs. remotely sensed data. Sommerfeld et al. [1994] and Williams et al. [1999b] have used initiated the use of geostatistics to analyze discharge measured with small arrays of snowmelt lysimeters and have suggested that preferential flowpaths may be organized on the scale of meters. Unfortunately, measurements of snowmelt are usually only collected at a few points [Harrington and Bales, 1998; Albert et al., 1999], hence it has not been possible to estimate variograms spanning many orders of magnitude in scale. Consequently, correlation lengths estimated from snow lysimeter data at the plot scale [Marsh and Woo, 1984a;b; Marsh, 1988; 1991; McGurk and Marsh, 1995] are unlikely to capture the large scale variability of snow melt that is due to large-scale topography and climatic conditions and, hence will underestimate the correlation lengths of the underlying true patterns of snowmelt in the region [Bloschl, 1999]. To understand the "scaling" of flow features in snow we need to develop better sampling strategies that are amenable to geostatistical analyses, including larger snowmelt lysimeter arrays and proxies for meltwater flowpaths such as remote sensing